Category Archives: nonequilibrium physics

Spectral signatures of symmetry-breaking dynamical phase transitions

R. Hurtado-Gutiérrez, P.I. Hurtado, C. Pérez-Espigares

Large deviation theory provides the framework to study the probability of rare fluctuations of time-averaged observables, opening new avenues of research in nonequilibrium physics. One of the most appealing results within this context are dynamical phase transitions (DPTs), which might occur at the level of trajectories in order to maximize the probability of sustaining a rare event. While the Macroscopic Fluctuation Theory has underpinned much recent progress on the understanding of symmetry-breaking DPTs in driven diffusive systems, their microscopic characterization is still challenging. In this work we shed light on the general spectral mechanism giving rise to continuous DPTs not only for driven diffusive systems, but for any jump process in which a discrete ℤn symmetry is broken. By means of a symmetry-aided spectral analysis of the Doob-transformed dynamics, we provide the conditions whereby symmetry-breaking DPTs might emerge and how the different dynamical phases arise from the specific structure of the degenerate eigenvectors. We show explicitly how all symmetry-breaking features are encoded in the subleading eigenvectors of the degenerate manifold. Moreover, by partitioning configuration space into equivalence classes according to a proper order parameter, we achieve a substantial dimensional reduction which allows for the quantitative characterization of the spectral fingerprints of DPTs. We illustrate our predictions in three paradigmatic many-body systems: (i) the 1D boundary-driven weakly asymmetric exclusion process (WASEP), which exhibits a particle-hole symmetry-breaking DPT for current fluctuations, (ii) the 3 and 4-state Potts model, which displays discrete rotational symmetry-breaking DPT for energy fluctuations, and (iii) the closed WASEP which presents a continuous symmetry-breaking DPT to a time-crystal phase characterized by a rotating condensate.

Phys. Rev. E 108, 014107 (2023); arXiv:2301.10262

Molecular hints of two-step transition to convective flow via streamline percolation

P.L. Garrido, P.I. Hurtado

Convection is a key transport phenomenon important in many different areas, from hydrodynamics and ocean circulation to planetary atmospheres or stellar physics. However its microscopic understanding still remains challenging. Here we numerically investigate the onset of convective flow in a compressible (non-Oberbeck-Boussinesq) hard disk fluid under a temperature gradient in a gravitational field. We uncover a surprising two-step transition scenario with two different critical temperatures. When the bottom plate temperature reaches a first threshold, convection kicks in (as shown by a structured velocity field) but gravity results in hindered heat transport as compared to the gravity-free case. It is at a second (higher) temperature that a percolation transition of advection zones connecting the hot and cold plates triggers efficient convective heat transport. Interestingly, this novel picture for the convection instability opens the door to unknown piecewise-continuous solutions to the Navier-Stokes equations.

Phys. Rev. E 106, 014144 (2022); arXiv:2207.09223

Active interaction switching controls the dynamic heterogeneity of soft colloidal dispersions

M. Bley, P.I. Hurtado, J. Dzubiella, A. Moncho-Jordá

We employ Reactive Dynamical Density Functional Theory (R-DDFT) and Reactive Brownian Dynamics (R-BD) simulations to investigate the dynamics of a suspension of active soft Gaussian colloids with binary interaction switching, i.e., a one-component colloidal system in which every particle stochastically switches at predefined rates between two interaction states with different mobility. Using R-DDFT we extend a theory previously developed to access the dynamics of inhomogeneous liquids to study the influence of the switching activity on the self and distinct part of the Van Hove function in bulk solution, and determine the corresponding mean squared displacement of the switching particles. Our results demonstrate that, even though the average diffusion coefficient is not affected by the switching activity, it significantly modifies the non-equilibrium dynamics and diffusion coefficients of the individual particles, leading to a crossover from short to long times, with a regime for intermediate times showing anomalous diffusion. In addition, the self-part of the van Hove function has a Gaussian form at short and long times, but becomes non-Gaussian at intermediates ones, having a crossover between short and large displacements. The corresponding self-intermediate scattering function shows the two-step relaxation patters typically observed in soft materials with heterogeneous dynamics such as glasses and gels. We also introduce a phenomenological Continuous Time Random Walk (CTRW) theory to understand the heterogeneous diffusion of this system. R-DDFT results are in excellent agreement with R-BD simulations and the analytical predictions of CTRW theory, thus confirming that R-DDFT constitutes a powerful method to investigate not only the structure and phase behavior, but also the dynamical properties of non-equilibrium active switching colloidal suspensions.

Soft Matter 18, 397 (2022); arXiv:2112.01191

Coupled activity-current fluctuations in open quantum systems under strong symmetries

D. Manzano, M.A. Martínez-García, P.I. Hurtado

Strong symmetries in open quantum systems lead to broken ergodicity and the emergence of multiple degenerate steady states. From a quantum jump (trajectory) perspective, the appearance of multiple steady states is related to underlying dynamical phase transitions (DPTs) at the fluctuating level, leading to a dynamical coexistence of different transport channels classified by symmetry. In this paper, we investigate how strong symmetries affect both the transport properties and the activity patterns of a particular class of Markovian open quantum system, a three-qubit model under the action of a magnetic field and in contact with a thermal bath. We find a pair of twin DPTs in exciton current statistics, induced by the strong symmetry and related by time reversibility, where a zero-current exchange-antisymmetric phase coexists with a symmetric phase of negative exciton current. On the other hand, the activity statistics exhibits a single DPT where the symmetric and antisymmetric phases of different but nonzero activities dynamically coexists. The presence of a strong symmetry under non-equilibrium conditions implies non-analyticities in the dynamical free energy in the dual activity-current plane, including an activity-driven current lockdown phase for activities below some critical threshold. Finally, we also study the effect of a symmetry-breaking, ergodicity-restoring dephasing channel on the coupled activity-current statistics for this model. Interestingly, we observe that while this dephasing noise destroys the symmetry-induced DPTs, the underlying topological symmetry leaves a dynamical fingerprint in the form of intermittent, bursty on/off dynamics between the different symmetry sectors.

New J. Phys. 23, 073044 (2021); arXiv:2104.13176

Simulations of transport in hard particle systems

P. I. Hurtado, P.L. Garrido

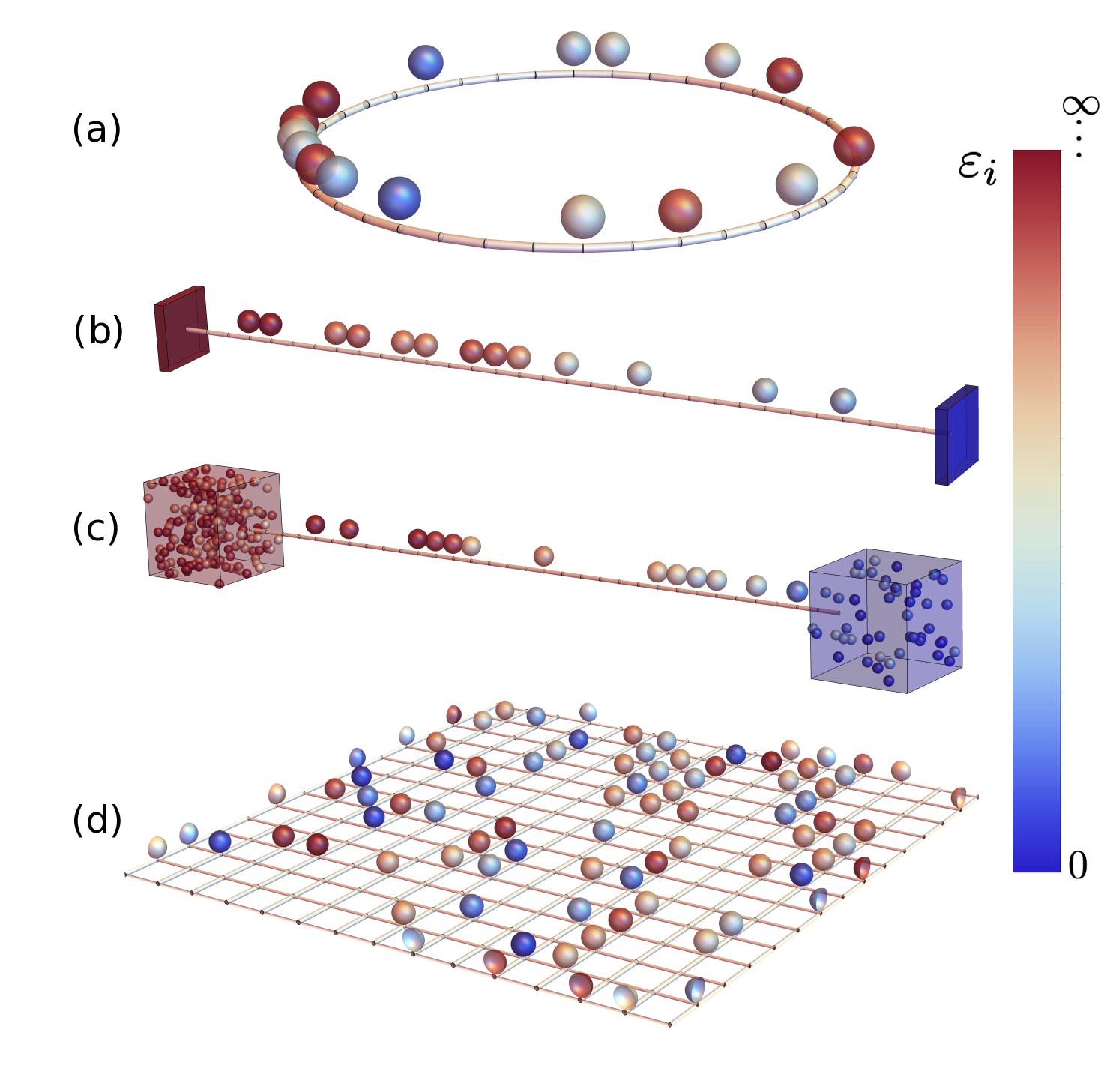
Hard particle systems are among the most successful, inspiring and prolific models of physics. They contain the essential ingredients to understand a large class of complex phenomena, from phase transitions to glassy dynamics, jamming, or the physics of liquid crystals and granular materials, to mention just a few. As we discuss in this paper, their study also provides crucial insights on the problem of transport out of equilibrium. A main tool in this endeavour are computer simulations of hard particles. Here we review some of our work in this direction, focusing on the hard disks fluid as a model system. In this quest we will address, using extensive numerical simulations, some of the key open problems in the physics of transport, ranging from local equilibrium and Fourier’s law to the transition to convective flow in the presence of gravity, the efficiency of boundary dissipation, or the universality of anomalous transport in low dimensions. In particular, we probe numerically the macroscopic local equilibrium hypothesis, which allows to measure the fluid’s equation of state in nonequilibrium simulations, uncovering along the way subtle nonlocal corrections to local equilibrium and a remarkable bulk-boundary decoupling phenomenon in fluids out of equilibrium. We further show that the the hydrodynamic profiles that a system develops when driven out of equilibrium by an arbitrary temperature gradient obey universal scaling laws, a result that allows the determination of transport coefficients with unprecedented precision and proves that Fourier’s law remains valid in highly nonlinear regimes. Switching on a gravity field against the temperature gradient, we investigate numerically the transition to convective flow. We uncover a surprising two-step transition scenario with two different critical thresholds for the hot bath temperature, a first one where convection kicks but gravity hinders heat transport, and a second critical temperature where a percolation transition of streamlines connecting the hot and cold baths triggers efficient convective heat transport. We also address numerically the efficiency of boundary heat baths to dissipate the energy provided by a bulk driving mechanism. As a bonus track, we depart from the hard disks model to study anomalous transport in a related hard-particle system, the $1d$ diatomic hard-point gas. We show unambiguously that the universality conjectured for anomalous transport in $1d$ breaks down for this model, calling into question recent theoretical predictions and offering a new perspective on anomalous transport in low dimensions. Our results show how carefully-crafted numerical simulations of simple hard particle systems can lead to unexpected discoveries in the physics of transport, paving the way to further advances in nonequilibrium physics.

J. Stat. Phys. 180, 474 (2020)

Building continuous time crystals from rare events

R. Hurtado-Gutiérrez, F. Carollo, C. Pérez-Espigares, P. I. Hurtado

Symmetry-breaking dynamical phase transitions (DPTs) abound in the fluctuations of nonequilibrium systems. Here we show that the spectral features of a particular class of DPTs exhibit the fingerprints of the recently discovered time-crystal phase of matter. Using Doob’s transform as a tool, we provide a mechanism to build classical time-crystal generators from the rare event statistics of some driven diffusive systems. An analysis of the Doob’s smart field in terms of the order parameter of the transition then leads to the time-crystal lattice gas (tcLG), a model of driven fluid subject to an external packing field which presents a clear-cut steady-state phase transition to a time-crystalline phase characterized by a matter density wave which breaks continuous time-translation symmetry and displays rigidity and long-range spatio-temporal order, as required for a time crystal. A hydrodynamic analysis of the tcLG transition uncovers striking similarities, but also key differences, with the Kuramoto synchronization transition. Possible experimental realizations of the tcLG in colloidal fluids are also discussed.

Phys. Rev. Lett. 125, 160601 (2020); arXiv:1912.02733

The kinetic exclusion process: A tale of two fields

C. Gutiérrez-Ariza, P. I. Hurtado

We introduce a general class of stochastic lattice gas models, and derive their fluctuating hydrodynamics description in the large size limit under a local equilibrium hypothesis. The model consists in energetic particles on a lattice subject to exclusion interactions, which move and collide stochastically with energy-dependent rates. The resulting fluctuating hydrodynamics equations exhibit nonlinear coupled particle and energy transport, including particle currents due to temperature gradients (Soret effect) and energy flow due to concentration gradients (Dufour effect). The microscopic dynamical complexity is condensed in just two matrices of transport coefficients: the diffusivity matrix (or equivalently the Onsager matrix) generalizing Fick-Fourier’s law, and the mobility matrix controlling current fluctuations, which are coupled via a fluctuation-dissipation theorem. Interestingly, the positivity of entropy production in the system then leads to detailed constraints on the microscopic dynamics. We further demonstrate the Gaussian character of the noise terms affecting the local currents. The so-called kinetic exclusion process has as limiting cases two of the most paradigmatic models of nonequilibrium physics, namely the symmetric simple exclusion process of particle diffusion and the Kipnis-Marchioro-Presutti model of heat flow, making it the ideal testbed where to further develop modern theories of nonequilibrium behavior.

J. Stat. Mech. (2019) 103203; arXiv:1905.03142

Sampling rare events across dynamical phase transitions

C. Pérez-Espigares, P. I. Hurtado

Interacting particle systems with many degrees of freedom may undergo phase transitions to sustain atypical fluctuations of dynamical observables such as the current or the activity. This leads in some cases to symmetry-broken space-time trajectories which enhance the probability of such events due to the emergence of ordered structures. Despite their conceptual and practical importance, these dynamical phase transitions (DPTs) at the trajectory level are difficult to characterize due to the low probability of their occurrence. However, during the last decade advanced computational techniques have been developed to measure rare events in simulations of many-particle systems that allow for the first time the direct observation and characterization of these DPTs. Here we review the application of a particular rare-event simulation technique, based on cloning Monte Carlo methods, to characterize DPTs in paradigmatic stochastic lattice gases. In particular, we describe in detail some tricks and tips of the trade, paying special attention to the measurement of order parameters capturing the physics of the different DPTs, as well as to the finite-size effects (both in the system size and number of clones) that affect the measurements. Overall, we provide a consistent picture of the phenomenology associated with DPTs and their measurement.

Chaos 29, 083106 (2019); arXiv:1902.01276

Infinite family of universal profiles for heat current statistics in Fourier’s law

P. L. Garrido, P. I. Hurtado, N. Tizón-Escamilla

Using tools from large deviation theory, we study fluctuations of the heat current in a model of d-dimensional incompressible fluid driven out of equilibrium by a temperature gradient. We find that the most probable temperature fields sustaining atypical values of the global current can be naturally classified in an infinite set of curves, allowing us to exhaustively analyze their topological properties and to define universal profiles onto which all optimal fields collapse. We also compute the statistics of empirical heat current, where we find remarkable logarithmic tails for large current fluctuations orthogonal to the thermal gradient. Finally, we determine explicitly a number of cumulants of the current distribution, finding remarkable relations between them.

Phys. Rev. E 99, 022134 (2019); arXiv:1810.10778

Quantum systems in and out of equilibrium: Fundamentals, dynamics and applications

P.L. Garrido, P.I. Hurtado, D. Manzano, F. de los Santos

The special issue of European Physical Journal Special Topics has been finally published. It originated at the 14th Granada Seminar on Quantum Systems in and out of equilibrium: Fundamentals, dynamics and applications, which took place in 2017, from June 20 to June 23 in Granada, Spain. This edition was sponsored by the University of Granada through the Department of Electromagnetism and Physics of the Matter and the Faculty of Sciences, the Spanish Minister of Economy, Industry and Competitiveness, and the European Physical Society. There were in this edition a total of 57 lectures and 27 poster contributions covering quantum aspects of thermalization, quantum transport, quantum effects in condensed matter, biology, quantum computation, open quantum systems, quantum fluctuations and large deviations, and quantum thermodynamics.

Eur. Phys. J Special Topics 227, 201 (2018)